Table of Contents
Trigonometry is an important topic in Quantitative Aptitude section of Competitive Examination mainly in SSC, Railways and Defence. Trigonometry is a branch of mathematics that examines the relationships between the sides and angles of triangles. It has diverse applications in fields like satellite navigation, astronomy, and geography, particularly in determining distances using the triangulation method.
Trigonometry Formulas
Six trigonometric functions are available: Sin, Cos, Tan, Sec, Cosec, and Cot. The current length and angle may be derived with the use of trigonometric ratios. These 6 functions serve as the foundation for all trigonometry formulae, formula tricks, and problems. The specifics of trigonometry, including the formulae, trigonometry formula tricks, and problems, are available to aspirants.
Trigonometry-related questions can be found in a variety of competitive exams, including SSC, Railway, and others. You may get notes about formula trigonometry that are helpful for exams in this site. It will assist you in learning the fundamental trigonometry formulae. The complete list of trigonometry formulae for classes 10 and 11, as well as trigonometry formulas for classes 12 and 13, is provided below.
In order to solve numerous trigonometric puzzles and comprehend how triangle sides and angles relate to one another, trigonometry formulae are essential. We will go through some of the basic trigonometric formulae here:
- Pythagorean Theorem: The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. Mathematically, it can be represented as: a² + b² = c² where a and b are the lengths of the two legs of the triangle, and c is the length of the hypotenuse.
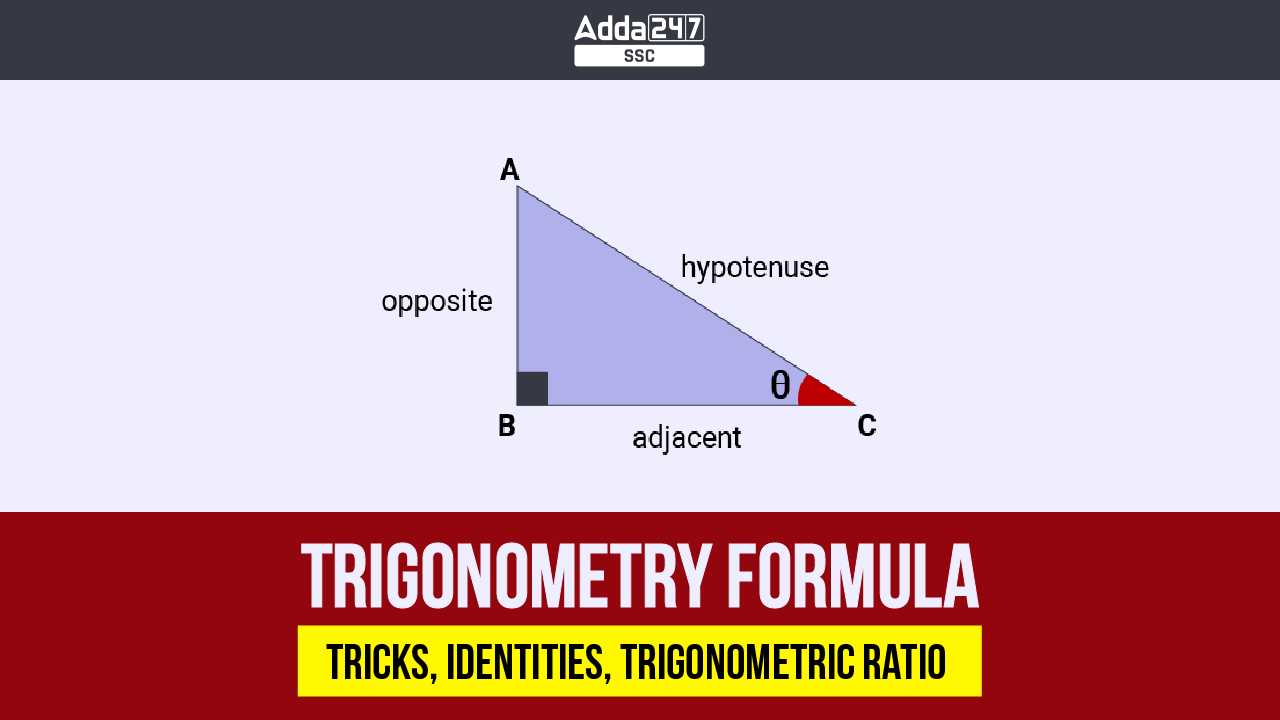
- Trigonometric Ratios: Trigonometric ratios relate the angles of a right triangle to the lengths of its sides. The primary trigonometric ratios are sine (sin), cosine (cos), and tangent (tan):
- Sine (sin)-> sinθ = Opposite/Hypotenuse
- Cosine (cos)-> cosθ = Adjacent/Hypotenuse
- Tangent (tan)-> tanθ = Opposite/Adjacent
- Reciprocal Trigonometric Ratios: The reciprocal trigonometric ratios are derived from the primary trigonometric ratios and relate the angles of a right triangle to the lengths of its sides:
- Cosecant (cosec)-> cosecθ = 1/sinθ
- Secant (sec)-> secθ = 1/cosθ
- Cotangent (cot)-> cotθ = 1/tanθ
- Angle Sum and Difference Formulas: These formulas express the trigonometric functions of the sum or difference of two angles in terms of the trigonometric functions of the individual angles:
- sin(A ± B) = sin(A)cos(B) ± cos(A)sin(B)
- cos(A ± B) = cos(A)cos(B) ∓ sin(A)sin(B)
- tan(A ± B) = (tan(A) ± tan(B))/(1 ∓ tan(A)tan(B))
- Double Angle Formulas: Double angle formulas relate the trigonometric functions of an angle to the trigonometric functions of double that angle:
- sin(2θ) = 2sin(θ)cos(θ)
- cos(2θ) = cos²(θ) – sin²(θ) = 2cos²(θ) – 1 = 1 – 2sin²(θ)
- tan(2θ) = 2tan(θ) / (1 – tan²(θ))
- Half Angle Formulas: Half angle formulas provide relationships between the trigonometric functions of an angle and the trigonometric functions of half that angle:
- sin(θ/2) = ±√[(1 – cos(θ)) / 2]
- cos(θ/2) = ±√[(1 + cos(θ)) / 2]
- tan(θ/2) = ±√[(1 – cos(θ)) / (1 + cos(θ))]
- Sum to Product and Product to Sum Formulas: These formulas convert sums or differences of trigonometric functions into products, and vice versa:
- Sum to Product Formulas:
- sin(A) + sin(B) = 2sin[(A + B)/2]cos[(A – B)/2]
- sin(A) – sin(B) = 2cos[(A + B)/2]sin[(A – B)/2]
- cos(A) + cos(B) = 2cos[(A + B)/2]cos[(A – B)/2]
- cos(A) – cos(B) = -2sin[(A + B)/2]sin[(A – B)/2]
- Product to Sum Formulas:
- sin(A)sin(B) = (1/2)[cos(A – B) – cos(A + B)]
- cos(A)cos(B) = (1/2)[cos(A – B) + cos(A + B)]
- sin(A)cos(B) = (1/2)[sin(A + B) + sin(A – B)]
- Sum to Product Formulas:
Just a handful of the basic trigonometric formulae are included here. These fundamentals serve as the foundation for several more complex formulae and identities. You’ll be able to tackle a variety of trigonometric issues with ease and precision once you’ve mastered these formulae.
Trigonometry Table
| Angles (In Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
| Angles (In Radians) | 0 | π/6 | π/4 | π/3 | π/2 | π | 3π/2 | 2π |
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
| tan | 0 | 1/√3 | 1 | √3 | ∞ | 0 | ∞ | 0 |
| cot | ∞ | √3 | 1 | 1/√3 | 0 | ∞ | 0 | ∞ |
| cosec | ∞ | 2 | √2 | 2/√3 | 1 | ∞ | -1 | ∞ |
| sec | 1 | 2/√3 | √2 | 2 | ∞ | -1 | ∞ | 1 |
Trigonometry Tricks
Trigonometry tricks are helpful techniques and shortcuts that can simplify calculations, solve problems more efficiently, and aid in memorizing key concepts. Here are some useful trigonometry tricks:
- Unit Circle: The unit circle is a valuable tool in trigonometry. By memorizing the coordinates of the angles (0°, 30°, 45°, 60°, and 90°) on the unit circle, you can quickly determine the values of sine, cosine, and tangent for these angles without using a calculator.
- Special Triangles: Special triangles, such as the 45-45-90 triangle and the 30-60-90 triangle, have well-defined ratios that make calculations easier. For the 45-45-90 triangle, the sides are in the ratio 1:1:√2, while for the 30-60-90 triangle, the sides are in the ratio 1:√3:2. These ratios can simplify calculations involving these angles.
- Symmetry: Trigonometric functions exhibit symmetry properties that can be exploited. For example, sine and cosecant have odd symmetry, while cosine and secant have even symmetry. Tangent and cotangent also have odd symmetry. Leveraging these symmetries can help simplify calculations by reducing the number of calculations required.
- Co-Function Identities: Co-function identities relate the trigonometric functions of complementary angles. Complementary angles are two angles that add up to 90 degrees (or π/2 radians). For example, sin(π/2 – θ) is equal to cos(θ), and cos(π/2 – θ) is equal to sin(θ). These identities can be useful for interchanging trigonometric functions and simplifying expressions.
- Radian-Degree Conversion: To convert between degrees and radians, you can use the fact that 180 degrees is equal to π radians. This conversion can be handy when working with both degrees and radians in a problem.
- Sum and Difference Formulas: The sum and difference formulas allow you to find the trigonometric values of the sum or difference of two angles. These formulas can help simplify complex trigonometric expressions. For example, the sine of the sum of two angles can be expressed as sin(A + B) = sin(A)cos(B) + cos(A)sin(B).
- Even-Odd Identities: Even-odd identities describe the symmetry of trigonometric functions. For example, sin(-θ) = -sin(θ) and cos(-θ) = cos(θ). These identities can be useful for evaluating trigonometric functions of negative angles.
- Periodicity: Trigonometric functions are periodic, meaning they repeat their values after certain intervals. For example, sine and cosine have a period of 2π radians or 360 degrees. Understanding the periodic nature of trigonometric functions can help simplify calculations and determine values for angles outside the standard range.
- Trigonometric Equations: When solving trigonometric equations, it is often helpful to use identities or manipulate the equation to simplify it into a more manageable form. This may involve factoring, canceling common terms, or applying trigonometric identities.
- Visualization and Drawing: Drawing triangles or visualizing the angles and sides of a problem can aid in understanding and solving trigonometric problems. Visualization can provide geometric insights and help identify relationships between different parts of a triangle or problem.
These trigonometry tips can improve problem-solving skills, speed up computations, and give shortcuts. To use trigonometry more effectively, it is crucial to practice and get familiar with these methods.
Trignometric Identities
Trigonometric identities are mathematical equations that establish relationships between trigonometric functions. These identities are derived from basic geometric principles and play a crucial role in simplifying expressions, verifying equations, and solving trigonometric problems. Here are some important trigonometric identities:
- Reciprocal Identities: Reciprocal identities express the reciprocal trigonometric functions in terms of their counterparts. These identities are useful for converting between trigonometric functions:
- csc(theta) = 1/sin(theta)
- sec(theta) = 1/cos(theta)
- cot(theta) = 1/tan(theta)
- Quotient Identities: Quotient identities relate the trigonometric ratios of one angle to the ratios of other trigonometric functions. These identities are helpful for expressing trigonometric functions in terms of each other:
- tan(theta) = sin(theta)/cos(theta)
- cot(theta) = cos(theta)/sin(theta)
- Pythagorean Identities: Pythagorean identities involve the Pythagorean Theorem and establish relationships between the squares of trigonometric functions. The most well-known Pythagorean identity is:
- sin^2(theta) + cos^2(theta) = 1
Other Pythagorean identities can be derived from this fundamental equation:
- 1 + tan^2(theta) = sec^2(theta)
- 1 + cot^2(theta) = csc^2(theta)
- Co-Function Identities: Co-function identities relate the trigonometric functions of complementary angles. Complementary angles are two angles whose sum is 90 degrees or π/2 radians:
- sin(π/2 – theta) = cos(theta)
- cos(π/2 – theta) = sin(theta)
- tan(π/2 – theta) = cot(theta)
- cot(π/2 – theta) = tan(theta)
- sec(π/2 – theta) = csc(theta)
- csc(π/2 – theta) = sec(theta)
These identities can be useful for simplifying expressions involving complementary angles.
- Even-Odd Identities: Even-odd identities describe the symmetry properties of trigonometric functions. The even-odd identities are as follows:
- sin(-theta) = -sin(theta)
- cos(-theta) = cos(theta)
- tan(-theta) = -tan(theta)
- csc(-theta) = -csc(theta)
- sec(-theta) = sec(theta)
- cot(-theta) = -cot(theta)
These identities show that the sine, tangent, and cotangent functions are odd functions, while the cosine, secant, and cosecant functions are even functions.
- Sum and Difference Identities: Sum and difference identities allow us to express the trigonometric functions of the sum or difference of two angles in terms of the functions of the individual angles. Some of the important sum and difference identities are:
- sin(A ± B) = sin(A)cos(B) ± cos(A)sin(B)
- cos(A ± B) = cos(A)cos(B) ∓ sin(A)sin(B)
- tan(A ± B) = (tan(A) ± tan(B))/(1 ∓ tan(A)tan(B))
These identities are useful in simplifying trigonometric expressions and solving trigonometric equations involving sums or differences of angles.
- Double Angle Identities: Double angle identities relate the trigonometric functions of an angle to the trigonometric functions of double that angle. These identities are derived from the sum and difference identities. Some of the key double angle identities are:
- sin(2theta) = 2sin(theta)cos(theta)
- cos(2theta) = cos^2(theta) – sin^2(theta) = 2cos^2(theta) – 1 = 1 – 2sin^2(theta)
- tan(2theta) = 2tan(theta)/(1 – tan^2(theta))
Double angle identities are particularly useful for simplifying expressions involving double angles.
Just a handful of the crucial trigonometric identities are listed here. You may simplify trigonometric expressions, check equations, and resolve a variety of trigonometric issues by applying these identities and comprehending their relationships. You will become more adept at trigonometry if you put these identities to use and get familiar with them.
Trigonometric Ratio
Trigonometric ratios relate the angles of a right triangle to the lengths of its sides. Understanding these ratios is fundamental in trigonometry. Here are the primary trigonometric ratios:
- Sine (sin): Sine is the ratio of the length of the side opposite the angle (O) to the length of the hypotenuse (H) in a right triangle. Mathematically, it can be expressed as: sin(theta) = O/H
- Cosine (cos): Cosine is the ratio of the length of the side adjacent to the angle (A) to the length of the hypotenuse (H) in a right triangle. Mathematically, it can be expressed as: cos(theta) = A/H
- Tangent (tan): Tangent is the ratio of the length of the side opposite the angle (O) to the length of the side adjacent to the angle (A) in a right triangle. Mathematically, it can be expressed as: tan(theta) = O/A
It’s important to note that these ratios are specific to right triangles and only applicable within the range of 0 to 90 degrees (or 0 to π/2 radians).
Additional Trigonometric Ratios:
- Cosecant (csc): Cosecant is the reciprocal of sine. It is defined as the ratio of the length of the hypotenuse (H) to the length of the side opposite the angle (O) in a right triangle. csc(theta) = 1/sin(theta) = H/O
- Secant (sec): Secant is the reciprocal of cosine. It is defined as the ratio of the length of the hypotenuse (H) to the length of the side adjacent to the angle (A) in a right triangle. sec(theta) = 1/cos(theta) = H/A
- Cotangent (cot): Cotangent is the reciprocal of tangent. It is defined as the ratio of the length of the side adjacent to the angle (A) to the length of the side opposite the angle (O) in a right triangle. cot(theta) = 1/tan(theta) = A/O
These trigonometric ratios are essential for calculating unknown angles or side lengths in a right triangle, as well as for solving various trigonometric problems. They provide a mathematical relationship between the angles and sides of a triangle, allowing for precise calculations and analysis.
Trigonometric ratios also have many practical applications beyond pure mathematics. They are utilized in fields such as physics, engineering, surveying, and navigation. By understanding these ratios, you can determine angles, distances, heights, and other relevant measurements in real-world scenarios.
Important Concept to Solve a Specific Type of Question
If A + B = 90°
Results that are true always :
(i) sin A. sec B = 1 or sin A = cos B
(ii) cos A. cosec B = 1 or sec A = cosec B
(iii) tan A. tan B = 1 or tan A = cot B
(iv) cot A. cot B = 1
(v) sin²A + sin² B = 1
(vi) cos² A + cos² B = 1
Important Trigonometry Formula for Sum and Difference Of Two Angles
Trigonometry formulas for the sum and difference of two angles play a crucial role in simplifying expressions and solving trigonometric equations. Here are some important formulas for the sum and difference of two angles:
- Sum of Two Angles:
- sin(A + B) = sin(A)cos(B) + cos(A)sin(B)
- cos(A + B) = cos(A)cos(B) – sin(A)sin(B)
- tan(A + B) = (tan(A) + tan(B)) / (1 – tan(A)tan(B))
- Difference of Two Angles:
- sin(A – B) = sin(A)cos(B) – cos(A)sin(B)
- cos(A – B) = cos(A)cos(B) + sin(A)sin(B)
- tan(A – B) = (tan(A) – tan(B)) / (1 + tan(A)tan(B))
These formulas can be derived using the identities and properties of trigonometric functions.
- Co-Function Formulas: Co-function formulas express the trigonometric functions of complementary angles in terms of each other. The complementary angle of theta is (π/2) – theta. Using these formulas, we can derive the sum and difference formulas for sine and cosine:
- sin(A + B) = cos(A’ – B’) = cos(A’)cos(B’) – sin(A’)sin(B’)
- cos(A + B) = cos(A’)cos(B’) + sin(A’)sin(B’) = sin(A’ – B’) where A’ = (π/2) – A and B’ = (π/2) – B.
- Double Angle Formulas: Double angle formulas relate the trigonometric functions of an angle to the trigonometric functions of double that angle. By setting A = B in the sum and difference formulas, we obtain the double angle formulas:
- sin(2A) = 2sin(A)cos(A)
- cos(2A) = cos^2(A) – sin^2(A) = 2cos^2(A) – 1 = 1 – 2sin^2(A)
- tan(2A) = (2tan(A)) / (1 – tan^2(A))
These formulas are useful when dealing with angles that are twice the size of a given angle.
- Half Angle Formulas: Half angle formulas express the trigonometric functions of an angle in terms of half that angle. These formulas can be derived from the double angle formulas:
- sin(A/2) = ±√[(1 – cos(A)) / 2]
- cos(A/2) = ±√[(1 + cos(A)) / 2]
- tan(A/2) = ±√[(1 – cos(A)) / (1 + cos(A))]
The ± sign indicates that there are two possible values for the functions, depending on the quadrant in which the angle lies.
These formulas for the sum and difference of two angles are essential in various trigonometric calculations, simplifying expressions, and solving trigonometric equations. Understanding and applying these formulas can greatly enhance your proficiency in trigonometry and enable you to tackle a wide range of problems involving angles.
Trigonometry Formulas For Tangent
Trigonometry formulas for tangent (tan) involve expressing the tangent of an angle in terms of other trigonometric functions. Here are some important formulas for tangent:
- Tangent Definition: tan(theta) = sin(theta) / cos(theta) This formula defines tangent as the ratio of the sine of an angle to the cosine of the same angle.
- Pythagorean Identity: sin^2(theta) + cos^2(theta) = 1 Dividing both sides of the equation by cos^2(theta), we get: tan^2(theta) + 1 = sec^2(theta) This formula relates the tangent to the secant of an angle.
- Tangent in Terms of Sine and Cosine: tan(theta) = sin(theta) / cos(theta) This is the basic definition of tangent.
- Reciprocal Identity: cot(theta) = 1 / tan(theta) This formula shows the reciprocal relationship between the tangent and cotangent functions.
- Tangent of a Sum or Difference of Angles: tan(A + B) = (tan(A) + tan(B)) / (1 – tan(A)tan(B)) tan(A – B) = (tan(A) – tan(B)) / (1 + tan(A)tan(B)) These formulas express the tangent of the sum or difference of two angles in terms of the tangents of the individual angles.
- Tangent of Half-Angle: tan(theta/2) = (1 – cos(theta)) / sin(theta) This formula relates the tangent of half an angle to the cosine and sine of the original angle.
- Tangent of Double Angle: tan(2theta) = 2tan(theta) / (1 – tan^2(theta)) This formula expresses the tangent of a double angle in terms of the tangent of the original angle.
- Tangent of Triple Angle: tan(3theta) = (3tan(theta) – tan^3(theta)) / (1 – 3tan^2(theta)) This formula calculates the tangent of a triple angle using the tangent of the original angle.
- Tangent of Difference of Squares: tan(A + B)tan(A – B) = [tan(A) + tan(B)][tan(A) – tan(B)] This formula relates the tangent of the sum and difference of two angles to their individual tangents.
These formulas provide valuable insights into the properties of tangent and allow for calculations involving angles and trigonometric functions. They can be used to simplify expressions, solve trigonometric equations, and derive relationships between different angles. Familiarizing yourself with these formulas will enhance your understanding of trigonometry and enable you to solve a wide range of problems involving tangent.
Trigonometry Formulas List
- Pythagorean Theorem:
- a² + b² = c² This formula relates the lengths of the sides of a right triangle, where a and b are the lengths of the two legs and c is the length of the hypotenuse.
- Trigonometric Ratios:
- Sine (sin): sin(theta) = opposite/hypotenuse
- Cosine (cos): cos(theta) = adjacent/hypotenuse
- Tangent (tan): tan(theta) = opposite/adjacent These ratios define the relationship between the angles and sides of a right triangle.
- Reciprocal Trigonometric Ratios:
- Cosecant (csc): csc(theta) = 1/sin(theta)
- Secant (sec): sec(theta) = 1/cos(theta)
- Cotangent (cot): cot(theta) = 1/tan(theta) These ratios are the reciprocals of the sine, cosine, and tangent functions.
- Trigonometric Identities:
- Pythagorean Identity: sin²(theta) + cos²(theta) = 1
- Reciprocal Identities: csc(theta) = 1/sin(theta), sec(theta) = 1/cos(theta), cot(theta) = 1/tan(theta)
- Quotient Identities: tan(theta) = sin(theta)/cos(theta), cot(theta) = cos(theta)/sin(theta)
- Co-Function Identities: sin(π/2 – theta) = cos(theta), cos(π/2 – theta) = sin(theta), tan(π/2 – theta) = cot(theta), csc(π/2 – theta) = sec(theta), sec(π/2 – theta) = csc(theta), cot(π/2 – theta) = tan(theta)
- Even-Odd Identities: sin(-theta) = -sin(theta), cos(-theta) = cos(theta), tan(-theta) = -tan(theta), csc(-theta) = -csc(theta), sec(-theta) = sec(theta), cot(-theta) = -cot(theta)
- Sum and Difference Formulas:
- sin(A ± B) = sin(A)cos(B) ± cos(A)sin(B)
- cos(A ± B) = cos(A)cos(B) ∓ sin(A)sin(B)
- tan(A ± B) = (tan(A) ± tan(B))/(1 ∓ tan(A)tan(B))
- Double Angle Formulas:
- sin(2θ) = 2sinθcosθ
- cos(2θ) = cos²θ – sin²θ = 2cos²θ- 1 = 1 – 2sin²θ
- tan(2θ) = 2tanθ / (1 – tan²θ)
- Half Angle Formulas:
- sin(θ/2) = ±√[(1 – cosθ) / 2]
- cos(θ/2) = ±√[(1 + cosθ) / 2]
- tan(θ/2) = ±√[(1 – cosθ) / (1 + cosθ)]
- Product to Sum Formulas:
- sin(A)sin(B) = (1/2)[cos(A – B) – cos(A + B)]
- cos(A)cos(B) = (1/2)[cos(A – B) + cos(A + B)]
- sin(A)cos(B) = (1/2)[sin(A + B) + sin(A – B)]
- Sum to Product Formulas:
- sin(A) + sin(B) = 2sin[(A + B)/2]cos[(A – B)/2]
- sin(A) – sin(B) = 2cos[(A + B)/2]sin[(A – B)/2]
- cos(A) + cos(B) = 2cos[(A + B)/2]cos[(A – B)/2]
- cos(A) – cos(B) = -2sin[(A + B)/2]sin[(A – B)/2]
These are just some of the important trigonometry formulas. There are many more advanced formulas and identities, but these should give you a solid foundation for working with trigonometry.
Trigonometry Maximum & Minimum Value
In trigonometry, the maximum and minimum values of trigonometric functions depend on the range of the angles being considered. Here are the maximum and minimum values for the primary trigonometric functions:
- Sine (sin):
- Maximum value: The sine function reaches its maximum value of 1 at 90 degrees (π/2 radians).
- Minimum value: The sine function reaches its minimum value of -1 at 270 degrees (3π/2 radians).
- Cosine (cos):
- Maximum value: The cosine function reaches its maximum value of 1 at 0 degrees (0 radians).
- Minimum value: The cosine function reaches its minimum value of -1 at 180 degrees (π radians).
- Tangent (tan):
- There is no maximum or minimum value for the tangent function since it is unbounded. As the tangent function approaches certain values of the angle, it tends towards positive or negative infinity.
It’s important to note that these maximum and minimum values apply to the standard range of angles (0 to 360 degrees or 0 to 2π radians). If angles outside this range or specific restrictions are given, the maximum and minimum values may differ.
Additionally, it’s worth mentioning that the maximum and minimum values can change depending on the units used (degrees or radians) and whether the angles are measured in the positive or negative direction.
Understanding the maximum and minimum values of trigonometric functions helps in analyzing and graphing these functions, solving trigonometric equations, and determining the behavior of angles within specific intervals.
Also/-
Maximum & Minimum Value
Minimum Maximum
- sin θ, cos θ [odd power] –1 +1
- sin θ, cos θ [even power] 0 +1
- tan θ, cot θ [odd power] –∞ +∞
- tan θ, cot θ [even power] 0 +∞
- sec θ, cosec θ [odd power] –∞ +∞
- sec θ, cosec θ [even power] +1 +∞



 Time and Work Notes, Simple Tricks To So...
Time and Work Notes, Simple Tricks To So...
 RRB NTPC Maths Previous Year Exam Questi...
RRB NTPC Maths Previous Year Exam Questi...
 SSC CGL Maths Syllabus 2024 for Tier 1 a...
SSC CGL Maths Syllabus 2024 for Tier 1 a...


